Breakthrough Thinking
Editor | On 01, Mar 2002
By: Larry Ball
larry.ball@honeywell.com
480-592-5919
Breakthrough Thinking This paper explains another article in this issue of The TRIZ Journal entitled Breakthrough Thinking , which presents a sequencing of TRIZ tools in a linear solution process. This material is available to individual readers for use in their own TRIZ studies. Those who seek to use the material for class instruction or other uses are requested to contact the author. While the author’s company has given him permission to publish TRIZ articles and solution processes and communicate with the TRIZ community, it does not make direct or implied endorsement of any of the author’s publications or communications.
Readers are encouraged to print out Breakthrough Thinking and place it into a binder. Tabbing is included to assist navigation. Each tab corresponds to one of the six top-level steps shown on the front of the binder.

Binder
Several levels of the class are preferred to match the students capabilities and interests. The highest level of the solution process is presented here.
This work is the result of ten years of classes, personal instruction from TRIZ experts, research, teaching and application and represents dozens of rewritings. It centers around the concept of functions.
Sequencing of TRIZ Tools One of the purposes of presenting this article is to introduce a train of study that may be productive in moving TRIZ to a new level of science. The current TRIZ tool-sets grossly overlap each other. This overlap makes it difficult to know which tool is the most appropriate for a given application. When the tool steps are broken out and regrouped into relevant groupings, patterns emerge that were not visible before. This method of investigation can be illustrated by the number patterns in the example below.
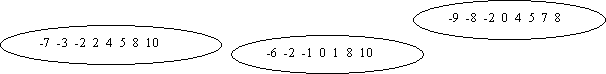
Each of the groupings represents a TRIZ tool such as S-field Analysis or 40 Principles. Note that there is over-lap between the groups where numbers are repeated. Although each number group is placed in sequence from lowest to highest, no patterns emerge. Without patterns to guide us, it is difficult the see what is missing. Now extract the numbers from the groups and reassemble them into groups of positive even numbers, positive odd numbers, negative even numbers and negative odd numbers as shown below.
![]()
In the context of these groupings, patterns emerge. For instance, we note that -4 is missing from the negative even numbers, while +6 is missing from the positive even numbers.
The material presented in Breakthrough Thinking is the result of reassembling the standard tool-sets into the relevant steps of a solution procedure. Care was taken to sequence the steps to ensure that in order to perform a given step, previous steps must be performed, or their output assumed. For example, in order to resolve a contradiction, certain key pieces of information must be known. These pieces of information are either developed in previous steps or they are unconsciously assumed. As a result, the solution process follows natural decision points, which branch to multiple solutions.
It is recognized that some well-known and useful tools have not yet been included. The reader is invited to make suggestions for improvement. Requests for the original PowerPointÃ’ presentation will be considered, should the reader be interested in arranging the steps in a more preferred sequence for personal use.
Goals of the Solution process The form of the solution process has been guided by the following goals:
AdjustabilityThe solution process should be teachable at a variety of levels to suit the capability and interest of the student. The top six steps are always taught, but sub-steps are simplified or eliminated and then added as the student gains greater mastery. This method of teaching is widely used to teach many subjects from music to mathematics. The focus should be on gradually increasing the ability of students while allowing success during the learning process.
Focus on Functions Functions are rapidly becoming the language of problem- solving in many disciplines. In this problem-solution process, virtually all steps revolve around the concept of functions.
Simple Nomenclature As with many disciplines, the nomenclature of TRIZ is often difficult to learn. One goal of the solution-process is to make the nomenclature fit ideas that the student is already familiar with. For instance, “Dynamism†is changed to “Make Adjustable,†and “Local Quality†is changed to “Make Non-uniform.†A certain amount of new nomenclature is unavoidable, and care should be taken to introduce it at higher levels of the class so as not to intimidate beginning students.
Smaller Steps Most beginning students are baffled by the seemingly “obvious†target- solutions presented in TRIZ literature. Many of these solutions are only obvious after the fact and represent large jumps in intuition. Some teachers may feel that these large jumps are a testament to the power of TRIZ and will try to impress the student with them. Unfortunately, many students are discouraged that such solutions are not as obvious to them. One goal of this solution-process is to decrease the step size, so that solutions are the result of several smaller steps rather than a few major leaps.
Visualization Along with the concept of “smaller steps†is the idea that solutions need to be visualized in order to become reality. Each step should aid the practitioner in visualizing a final solution. Some may feel that elegance or compactness is sacrificed by expanding classical TRIZ steps, but the goal must always be to make the solution more easily visualized.
Completeness of Solution The term “solution†means different things to different people. For the sake of this solution-process, a solution is a sketch that someone could work from to design hardware. No difficult contradictions or problems would remain to be solved. Simply pointing out a physical phenomenon that might be used to solve a problem would not, in this context, be considered a solution, since difficult challenges would inevitably be encountered before a practical solution was evident.
Functional Nomenclature It is recognized that the proliferation of TRIZ terms is objectionable and makes it difficult for the new student to translate between different authors. Sometimes different terms are used to mean the same thing. In order for the reader to “translate†while reading Breakthrough Thinking, a consistent nomenclature will be established.
A System is a collection of objects which provide a function.
In this text and in Breakthrough Thinking, the physical element which is acted on will be referred to as the Product. In other texts, it may be referred to as the object or artifact. The object which acts on the Product is referred to as the Tool.
The action or change which the tool performs on the product will be referred to as the Modification. In some texts, this is referred to as the action. It is usually a verb. The use of the term “Modification†will be new to many readers, however it is used to stress the requirement that the action verb must describe a change or maintenance. This is sometimes difficult for new students to grasp. Students of the author are encouraged, in the beginning, to use a longhand form of the modification. The longhand form begins with “Change†or “Maintainâ€. For example, we can describe the action that occurs between a tool “liquid†and a product “thermometer†which is immersed in the liquid. The short form of the modification is “ heats†or “cools.†The longhand form of the modification would be “Changes the temperature.â€
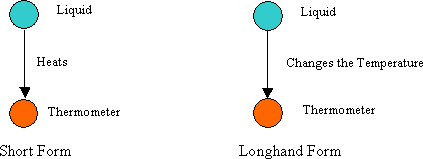
The use of the term “modification†helps the student to understand that the tool and product must be physical elements. It also helps the student to correctly describe “confusing functions,†such as how paint protects wood. New students often say “ Paint-protects-woodâ€. While the word “Protects†is a verb, it is not a modification as it does not describe a change to the wood. Insistence on using the word “Protects†will hamper the problem solver in later steps. The longhand form immediately encourages the student to correctly write “Paint-maintains the location of —moisture†and “wood-maintains the position of —paint.†The short version now becomes “ paint — stops– moisture†and “wood-holds-paintâ€. Once the long-hand form is firmly entrenched, the student can usually revert back to the short form of the modification for brevity.
The modification to the product is delivered by an Effect. The term “Effect†is an artificial convention, introduced by TRIZ practitioners that allows us to group physical phenomenon into recognizable groupings. An example of an Effect would be “capillary action.†The Effect of capillary action actually arises from complex physical interactions involving several different fields at the atomic level. However, most people readily recall the phenomenon of capillary action from demonstrations of liquids rising in tubes. TRIZ literature contains tables of these Effects which are very useful.
A Field, as described in TRIZ literature, is another artificial grouping convention which allows us to talk about different manifestations of fundamental fields as recognizable groups such as vibration, electromagnetic radiation, gravity, mechanical stress and pressure. Some of these “fields†such as Odor & Taste may seem a little off-beat. Effects, as described above, are delivered by one or several objects and their associated fields.
In summary, the Tool delivers the Effect, which delivers the Modification to the Product.
Solution-process Steps Following are explanations of the solution-process steps as described in Breakthrough Thinking.
Set High GoalsThe important outputs of this step are: a clarification of what the system is; what we will and will not try to change within the super-system; the main disadvantage that we want to overcome; what we want to happen to the system and super-system as a result of the changes; and the maximum system penalties which we will allow, such as cost.
Choose or Create the Subject System Identifying a system that needs improvement helps to focus the problem solver and to more clearly determine system disadvantages. If the subject system does not currently exist, it is acceptable to guess at or create a surrogate system.
Determine Customer Requirements and System Disadvantages Identify customers and then work with them to determine system requirements and current disadvantages.
It is important to verify that a disadvantage really exists. This is especially true in the area of reliability improvement. Shop data can be misleading. For instance, a repair shop sees a certain product failure over and over and concludes that the product is unreliable. What the repairman does not see is all of the units that operate successfully for long periods of time, making the overall reliability very high.
Model the Current Technical System or Process Use Function Analysis to model the system or process. Although modeling the current process will aid in the process of determining what is causing the disadvantages, this is not the main tool for cause-effect analysis. This step helps to determine what the system really is and what the penalties are for delivering the modification to the product. (Note that a function-like structure for disassociation and combination are introduced in the process diagrams. Classical function modeling is clumsy at describing these spontaneous chemical processes).
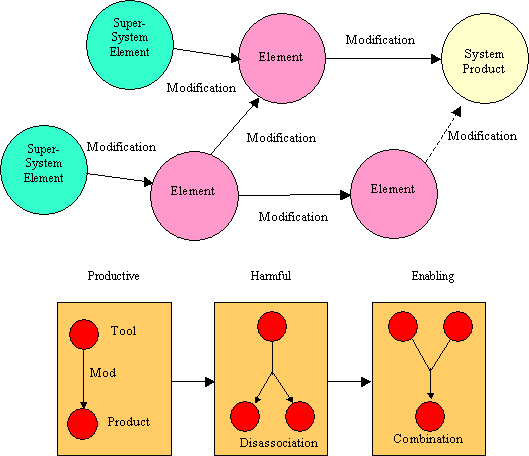
Determine Element or Process Step Values Knowing the penalties for delivering the required function to the system product will influence our goals.
Set a High Bar It is extremely important to set high goals. A goal is only high when we sufficiently constrain the system penalties (such as cost–Anyone can make a system improvement that costs a lot and requires increasing complexity). Because TRIZ practitioners gain the confidence to tackle harder and harder problems, they are not hesitant to set high goals. High goals can galvanize the solution effort, especially when working with groups.
Identifying Critical Functions (Cause & Effect) When a system has a problem or disadvantage, usually there are chains of critical functions, where functions are linked to each other by cause or by requirement. If these functions can be discovered early in the problem solving process, the “right problem†can then be solved. One of the first steps in any problem solving methodology should be to identify the most important functions through some form of cause-and-effect analysis.
A common mistake is to assign cause-and-effect from armchair observations. Do your homework! Search the Internet. Read books on the subject. Talk to subject matter experts and weigh their words carefully. (There is a lot of misinformation even among experts). Get your hands dirty with experiments and microscopic observation. Develop theories, quantify them and verify them, even when you think the theory of operation is obvious. It is very common that new theories of operation will be developed. This step is usually the most time consuming, but it yields huge benefits if the appropriate time is taken.
The problem solver is encouraged to do five main things:
1) Form theories
2) Quantify theories
3) Verify theories
4) Identify controlling variables
5) Link causal relationships
Function Chains link causal relationships. This method, as shown in the solution process, is similar to the “Problem Formulation†step described in Systematic Innovation. Below is an example of the beginning of a Function Chain:
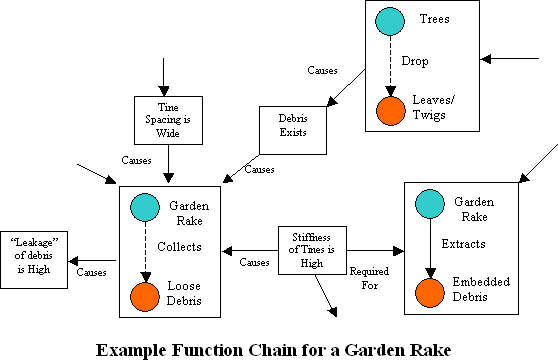
In this example we are considering why a common garden rake (with stiff tines) is poor at collecting loose debris. The independent variable of debris “leakage†is high. It occurs in the function of “garden rake collects loose debris†and is controlled by a number of independent variables such as tine spacing, existence of debris and stiffness of the tines. Note that a value is given to the dependent variable of leakage (high) and that values are given to the independent variables of spacing (wide), existence (exists), and stiffness (high). These values cause the dependent variable, leakage, to be high. In turn, each of these independent variables are either required to perform other useful functions or are designed-in for trivial reasons. For example, the stiff nature of the tines is required to perform another function, the extraction of embedded debris. The current shape of the tines may be unimportant.
Interesting contradictions become apparent. We note that the stiffness of the tines is high in order to extract embedded debris, but must be low in order to follow the contours of the earth and extract loose debris. There are other reasons that the tines must be stiff, such as for arranging soil. So, the tines must be stiff and flexible. Later we will consider how to resolve this contradiction.
The author encourages his students to use flow-charting software to make these diagrams as they can become complex and there is a need to arrange figures quite often.
Eliminate or Replace Parts Once the critical functions are determined, the question becomes “How can these functions be improved?†The improvement of a critical function is broken into two steps. The first step, Eliminate or Replace Parts, deals with determining what the function parts should be. Only after the elements are determined, will we concentrate on the next step, Improve to the Extreme, by boosting the function or removing flaws. Let us first consider Eliminating or Replacing parts to make the most ideal use of system resources. Each part (product, modification, Effect/Tool ) is considered in turn and the question is asked, “Do I want to eliminate or replace this with something more ideal?â€

Another name for the step Eliminate and Replace Parts might have been the Ideal Final Result, a familiar tool of classical TRIZ. The Ideal Final Result, is included in this step but not in its most familiar form, since it is broken up into smaller steps to aid visualization. The “Standard Solutions†of S-field analysis that deal with eliminating, redefining or replacing function parts are placed in this step. When combined with the concept of Ideal Final Result, this part of the solution process becomes very powerful. This is the opportunity to greatly reduce the system penalties by decreasing the number of elements and allowing elements take on more functions.
The solution path depends upon whether we are considering Useful, Harmful or Informing (measuring or detecting) Functions. This is because the initial focus changes. (Note that the tab system for the binder differentiates between these function types). For Useful Functions, the initial focus is on the modification of the product. For Harmful Functions, the initial focus is on the tool and modification. For Informing Functions, the initial focus is on the tool .
Useful Functions As mentioned, the initial focus for a useful function is the modification of the product. In essence, we are asking, “What do we really want to have happen to the product?†Many problem solvers skip over this step, unaware of their unconscious presumptions. In doing so, some of the most powerful solutions are ignored. Once we are firm on the most desirable outcome, we can ask how the modification to the product will be accomplished.
The Ideal Product The first question that we ask ourselves is “What product do we really want to modify?â€
Non-Transmission Product If the tool is currently operating on a transmission element, we consider bypassing the transmission and operating more directly on the product that the transmission operates on. This is consistent with the evolutionary law which states that transmission paths are shortened and the number of energy transformations are reduced. Take the example of a ships anchor. In functional language, the sea bed constrains or holds the anchor. The anchor holds or constrains the chain and the chain holds the ship. By realizing that the anchor and the chain are transmission paths to the ship, we now have a decision to make. Do we want to constrain the anchor, the chain or the ship directly? Each decision begins a different solution path.

Non-Existent Product Next, we ask if the product is normally considered waste. If it is, then we consider ways that it can be eliminated. Once the product is eliminated, the tool or system that performs the modification is no longer required. This changes the problem to that of removing the source or path of the product. For example, if there is no source for the leaves or there is no path for them to reach the lawn, then they do not need to be collected. Elimination of the product often leads to a direct contradiction in that the product must exist and must not exist.
Product Requires Little or No Modification Next, we look for ways that the product does not require the modification in the first place. Often there is something about the product that makes the modification necessary. If that one thing can be changed then the function may not be required. For example, the scales of a fish are often removed before consumption. If the scales were considered a delicacy, they would not require removing. The problem now becomes one of improving the texture and taste of scales.

Comes That Way Next, we consider a product that does not require the modification because it is already incorporated. Altshuller gives an example of the need to rapidly cut tubes formed by a rolling machine that processes flat metal stock. As the forming machine gets faster and faster, the cutting of the tubes becomes more difficult. The question is asked “What if the tube comes already cut?†Then no cutting will be required. Again, this method often leads to a contradiction. The tube comes in a cut condition, but it must not because it arrives as flat stock on rolls. (In Altshullers book, the contradiction is finally resolved by cutting the flat stock, but only partially. The final cut comes from a rapid pull of an electromagnet).

Other considerations in this section of Breakthrough Thinking are modification of the Minimum Part, Multiple Products, Biased Products and Diverse Products.
 The Ideal Modification In this section we ask “What is the modification that I really want to perform on the product?†(This assumes that the product still requires a modification). We are in effect replacing or redefining the modification requirement of the useful function. In an overall sense, we ask “If I could snap my fingers what would I want to have happen to the product?â€
The Ideal Modification In this section we ask “What is the modification that I really want to perform on the product?†(This assumes that the product still requires a modification). We are in effect replacing or redefining the modification requirement of the useful function. In an overall sense, we ask “If I could snap my fingers what would I want to have happen to the product?â€
Main Modification Our first task is to look into the future and ask what the final, final state of the product should be. The example is given of a fan “blade out†condition where rapidly rotating blades break off from their hub. This is an extremely harmful condition that sometimes occurs in jets and fan jet engines when the blades are struck by flying objects. Usually a “containment ring†is placed around the blades. As the blade fly off, they strike the containment ring, protecting other engine components and passengers. Now we ask “What is the modification to the broken blades that we really require?†Do we want to deflect the broken blade pieces or do we really want to completely absorb their energy?

It is important to take time with this step and ask “what do I really want to have happen?†Or, “If I could snap my fingers….What would happen?â€
The Inverse Every modification is performed relative to something else. For instance, the function may be to transport a passenger. We ask “in relation to what?†In this case, the passenger is being transported in relation to a store. The inverse would be to transport the store to the passenger. Is this possible? By considering the inverse, we may have discovered a more ideal product and modification.

Additional tests are given to determine whether the function is excessive or to focus our thinking on the minimum resources that are required to perform the modification (time, volume, and energy).
The Ideal Tool / Effect Finally, a tool with its accompanying Effects and Fields is required to deliver the modification. The Tool and Effect are considered together because we want to consider resources that are readily available, especially objects that are already part of the system or super-system and if possible, already act on the product.
Self Service Definition: a slight change to the product allows an existing external field which already acts on the product to deliver the required modification. No new Tool or field should purposely be introduced. Instead, the field should exist at some point during the product’s life-cycle but before the modification is actually required. Let us take a self cutting pie for example. We ask, “What fields does the pie experience during its making but before consumption?†(Page 10 contains a list of fields). We note that sometime during the life, the pie will experience a thermal field while it is being baked. We then ask, “what modification to the pie crust causes the crust to segment when it experiences this thermal field?â€

Abundant Native Fields Where Self Service requires a slight modification to the product to make use of an abundant native field, “Abundant Native Fields†requires a slight change to fields which already exist in the super-system and which may already act on the product. Again, no new Fields or Tool are required. On page 10 of Breakthrough Thinking is a list of fields. Usually, a couple of these fields will exist in the environment and already deliver the required modification to some slight degree. If such a field is identified, sometimes it can be boosted in the next solution step.
In the book Creativity as an Exact Science, an example is given of lightning rods being used to protect radio telescopes from lightening. The problem is that when metallic rods are constructed to safely conduct the electrons, the rods corrupt the radio signals from space. This example is often used to illustrate the concept of Ideal Final Result. Unfortunately, for many students, this conceptual leap is too great. Use of Abundant Native Fields provides a smaller step towards the Ideal final Result.
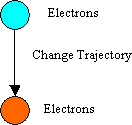
If we have been following the steps, we will have already recognized that the ideal product that is being modified is the electrons of the lightening bolt. The useful modification is to change their trajectory or to conduct the electrons.

Now, we search for a Tool to deliver this modification. The question is poised “Is there a tool in the system or super-system that already delivers the required modification, even poorly?†The answer is Yes, the air already conducts the electrons, though with little control.†Thus, a new solution path is created. In the next step, we will see how this function can be boosted to give more control to the trajectory of the conducted electrons.

If there were not a tool which already delivered the modification we would search for one in the vicinity by looking for abundant native fields. We would have asked, “what abundant native fields can change the trajectory of electrons.†Examining the table of fields found on page 10, we might recognize that electrostatic and magnetic fields have the potential to change the trajectory of electrons. Are these fields abundant? Yes, both are abundant but self- generated and poorly controlled. In other words, the electrons, themselves will deliver the modification by changing the trajectory of the electrons.
At this point, we may be scratching our heads over how air or electrons could be used to change the trajectory of air. That is expected because we are taking small steps toward the solution. The solution may not be apparent at this point. Take heart. In the next step Improve to the Extreme, we will examine how these functions are boosted to give a more complete solution.
Analogous Tools Several means for discovering new tools to perform the modification are considered, which invite the problem solver to consider how the modification is already delivered in other systems. Four types of analogous tools are considered. Nearby Analogous Tools, Analogous Tools, Mega-trend Analogous Tools, and Natural Analogous Tools.In order to understand the concept of analogous tools, the concept of, analogous products must first be understood.Analogous tools modify analogous products. An analogous product is one that requires the same modification as the product in question. For example, consider the removal of a sliver. We ask “What other products require the same modification as the sliver (removal) while firmly embedded in a much larger object?†Products such as nails and weeds come to mind. Nails and weeds are analogous to our sliver in the context of the modification (removal). Now, we consider the tools which deliver the modifications to these analogous products. Claws on crowbars and hammers remove nails from larger objects. Forked digging tools likewise remove weeds. Next we transfer the Tool or part of the Tool to the new situation. The final Tool is a mini-claw which removes the sliver.

Cheap Abundant Substances and Adjacent Elementsare methods to use objects in the system or super system to deliver the modification. The successful use of this method usually involves low-level fields (fields toward the top of the list of fields) which are more abundant, yet more difficult to control.
Table of EffectsThe table of effects is a good tool for finding new Effects to deliver the modification. First convert the modification into a form that most closely matches the modifications given in the Table. Then look for the suggestions in the Table. Breakthrough Thinking does not contain this table as it would be too large. The best tables are found in invention software.
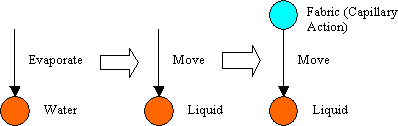
Patent Data Base The U.S. patent data base can be used from the internet. Do not expect the data base search tools to have the same search capabilities as an internet search engine. It takes time to learn effective search techniques, but the patent data base is a rich source of ways to deliver a modification, and it is still free.
Current ToolWhen all else fails, use the current tool. There is no great shame, this is a common solution path.
Harmful Functions Harmful functions have a different focus than useful functions. There are two ways to eliminate and replace function parts: Turning Harm to Good and Elimination.
Turning Harm to Good In America, there is a saying, “When life gives you lemons, make lemonade.†A harmful function, like a useful function, contains the same parts. There is Tool which delivers a harmful modification to a Product. In turning harm to good, we consider keeping the tool with its attendant fields and effects and replace the harmful modification with a useful one.
The Product of this new useful modification is usually the current product of the harmful function. For this reason, we first consider useful functions on the current product, the anti function and a useful variant of the harmful function.
Anti-function. If heating is the harmful modification, then the anti-function would be to cool.

Useful Variant of the harmful function: If a Tool wears the product then a useful variant of the harmful modification to “wear†is to “shape.â€

Once a useful modification is identified, then one of the five following methods is used to achieve this useful modification. Of the five methods, the two most important methods are to reverse the fields and to make adjustable. Almost any harmful function can be turned into a useful function by reversing the fields or making it adjustable.
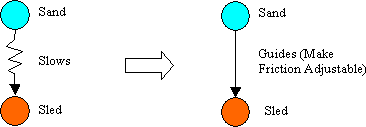
Reverse the Field or Action The easiest way to achieve the anti-function is to reverse the fields or the action. If the pressure is pushing the product one way, reverse the pressure field. Make something pull rather than push. If air is entraining contaminants, then make it expel the contaminants by reversing the pressure field.

Make Adjustable Perhaps the easiest way to achieve useful variant of the harmful modification is to make it adjustable. As stated, almost any harmful modification can be made useful by making it adjustable. Note that later we will make suggestions on how to make a useful modification adjustable. This involves identifying controlling variables of the interaction between the two elements and then making one or more of these adjustable.
Work WithOften, the useful modification will be performed at the same time as the harmful function. Because they are not in equilibrium, the harmful function predominated. For instance, wear is actually the process of removing and adding material. The amount that is removed is more than the amount added. If we can boost the useful component of this equilibrium state, then we can perform a useful function.
Incorporation Artists often use this technique to hide flaws in a work of art, especially if the medium does not allow “erasing.†One technique is to multiply the flaw in a useful way. For example, a blade cuts off a piece of plastic pipe. In the process, the pipe is deformed on one side. Although this flaw may not reduce the usefulness of the pipe, it is unsightly. This flaw is improved by multiply it around the whole pipe, thus creating a pleasant bevel. This bevel may now help to guide the pipe during installation.
Perform Accurately The final method for making a harmful modification useful is to perform it more accurately. Many harmful actions are harmful only because of the variation. The task that remains is to identify where or when the task should be performed precisely to make it useful. This method is a subset of making adjustable.
Elimination One of the most direct routes to improving a harmful function is to eliminate either the tool or product. Usually a contradiction results. When it does, we bypass the next step “Improve to the Extreme†and go directly to “Resolve the Conflict.â€
Non-Existent Tool This is the most commonly used and useful consideration. Since the tool harms the product, consider that it no longer exists. During the resolution of the conflict, two general paths are followed. Either resolve the contradiction or consider a new system where the Tool does not exist. Any useful function performed by the Tool must be performed by something else.

Non-Existent ProductSometimes a harmful modification is delivered to a product, which is not required in the system in the first place, such as a waste product. Consider removing the Product, its path or its source.

Informing Functions Although informing functions are useful functions, their beginning focus is on the tool and the variable in the tool that requires detection.
Ideal Variable that Requires Detection. What is the variable or feature of the Tool which requires detection? As with the ideal modification, some thought is required to determine this variable in an ideal manner. A longhand method can be used for clarity.

Ideal Tool In useful functions, we considered the ideal product. Here, we consider the ideal Tool using the same process steps. For instance, in useful functions, we considered products which required no modification. Here we consider tools which require no detection or measurement. There is nearly a one-to-one correspondence with useful functions.
Roundabout Variables Once we have determined the variable, we can consider means that allow us to detect or measure the variable indirectly.
Ideal Effect The Table of Effects has a special section on detection and measurement. Search this table to determine phenomenon which can be used to deliver the detection required.
Use of Markers One method which requires special consideration is the use of Markers. This includes both substance and field markers which can be detected by the product.

Ideal ProductAlthough not Shown, a product is required to be acted on by the tool. At this point, a more clear Useful Function can be drawn. With a clearer picture of the Informing Function, we are ready for the next step of improving this function to the extreme.

Improve to the Extreme (It is assumed, beyond this point ,that the Tool, Modification and Product will be preserved). Once we have eliminated or replaced function parts, there is usually something which is undesirable about the function. For instance, the performance of the function may require too much energy or time.
The focus in this step is to concentrate on this one function and its improvement. It will be improved to the extreme without consideration for anything else that might become worse. It is precisely this form of extreme thinking which allows the formation many useful contradictions.
We are about to create a diagram whose form is very useful and bears repeating. It includes the function, the improvement, the controlling variables and the contradiction. Improving the function to the extreme is performed in three steps:
Identify the Improvement (Dependant Variable) This step requires that we first determine the dependent variables of the flaw that we want to improve. In this case, we are considering the function of a rake to collect loose debris. Since we are using a garden rake rather than a leaf rake, the rake does not collect as much as we would like on each pass. We want to improve the “leakage†of debris past the tines. In doing this, we also consider the ideal outcome. In this case, “ideal outcome†means an enduring solution. If possible, we would like all of the debris to be collected on each pass.

Identify Controlling (Independent) Variables Next we determine the independent variables used to control the improvement. We can look at this several ways. Most people simply start with intuitive guesses. If we were going to write an equation, what would “leakage†be a function of? Each of the variables of the equation would be candidate variables which control the leakage of the rake. If we have gone to the trouble of developing models, we can often use input variables from these models. Without quantitative data, we can still consider variables that we know from experience or from crude experiments. On the function diagram, we write that leakage is a function of those things which we have identified through the given methods. We note that were the tine spacing closer, less debris would slip through. If the tines followed the unevenness of the ground, it would collect more efficiently. Also, if the ground were more flat, there would be no need to follow it so closely.

Having exhausted our own knowledge, we turn to the Table of Controlling Variables which are on the next nine pages of Breakthrough Thinking. Sub-steps are given with each controlling variable to make the identification of each Controlling Variable more clear.
It is noted that this table is mostly a derivative of the “Standard solutions.†That is because a large part of the Standard Solutions already deal with the control of functions. Since we have already used the Standard Solutions that deal with the replacement of function parts, and moved them forward in the thinking process, what remains are the controlling variables that can be used to improve the function. Indeed, almost every method for controlling a function can be found in the Standard Solutions.
In its original form the Standard Solutions contained solutions with the contradictions already removed. A notable case for this is the addition of internal, external, and environmental additives. In Contrast, the Table of Controlling Variables assumes that to improve the specific dependent variable, the whole tool or product is made of the required substance. This causes all kinds of problems. The removal of the contradiction is delayed in favor of creating a greater number of ways to resolve the contradiction than those included in the Standard Solutions.
Experience shows that using the Table of Controlling Variables will help the problem solver uncover several unanticipated ways to control the function. We note that the debris has uneven shapes and would be collected better if it were aligned more favorably with the tines. If there were less debris, there would be less leakage. If the debris shapes and sizes were more favorable, it would collect better. And, if the debris were light, the tines would have less tendency to roll over it.

Finally we consider each variable in turn and ask the question: What is this variable measured relative to? Consider changing this “relative to†variable in addition to the others. This is part of “Doing the Reverse.†If the ground were flexible or easily penetrated (soft), there would be no need for the tine flexibility. If the tines were instantaneously uneven to match the shape of the ground, there would be no need for the ground to be even. If the tines were heavy compared to the debris, it would have less tendency to roll over the debris.

Drive Variable Values to the ExtremeFinally, the variable values are driven to the extreme to improve function flaws. This is done without consideration for anything else that might get worse. Focusing this way is precisely what is needed to break psychological inertia.
When we drive a controlling variable to the extreme, something else usually gets worse, or it appears impossible. Thus we see that the variable must have the new value and it must not. Without noting the reason that the extreme value will not work, we include the anti-value in the diagram and thus capture the contradiction as a natural result of this step.

Infrequently, a variable can be pushed to the extreme without affecting anything else. If this happens, consider yourself lucky.
Sometimes we do not know why such a solution wouldn’t work. Fortunately, others are always on hand to point out the fallacy of our thinking. “That won’t work because….†Criticisms are often a good way to discover contradictions.
Resolve Contradictions While improving to the extreme, it is common to develop many contradictions. Each of these contradictions represents a new branch or solution path. Classical TRIZ considers three types of contradictions, Administrative, Technical and Physical.
The Administrative Contradiction states that there is a problem with an unknown solution. This form of contradiction informs us of little and is ignored here.
The Technical Contradiction states that as something improves something else gets worse. In the rake example, we could have stated that the tines needed to be flexible and stiff. No rational was given in the function diagram. In reality, the tines need to be flexible to collect and they need to be rigid in order to extract embedded debris from the ground. The resulting Technical Contradiction is: as collecting improves, extracting becomes worse. (No mention of tine stiffness is included).
The Physical Contradiction states that the value of a controlling variable must be either one value and an extremely different value referred to as the “anti-value†or “anti-property†(usually the opposite or null value). In the rake example, we developed several Physical Contradictions. For instance, the tines must be stiff and flexible.
In this text, Technical Contradictions are not articulated in favor of Physical Contradictions for the following reasons:
An acknowledgement of at least one thing that gets worse, when we drive a controlling variable to the extreme, is usually sufficient.
Technical contradictions arise from physical contradictions and not the reverse. In other words, Physical Contradictions are not discovered by peeling away the layers of the technical contradiction, as implied by some texts. Note that in the example of the rake, we first drive the controlling variable of tine stiff ness to the extreme and then look around to see what got worse. (It is interesting to note that some algorithms for creating the Technical Contradiction inadvertently create a Physical Contradiction first and then deduce the Technical Contradiction).
Most algorithms strive to develop the Technical Contradiction. Actually, for some Physical Contradictions, several Technical Contradictions can be formed. In other words, there may be several reasons that an object cannot be both hot and cold. Since we have shown that it is easy to develop multiple Physical Contradictions, the resulting number of Technical Contradictions increases the complexity of the solution process.
Creation of the Technical Contradiction does not increase the ability of the problem solver to directly visualize the solution. Although it appears that the problem is made more difficult by forming the Physical Contradiction, the problem solver is brought much closer to the solution because of the enhanced ability to visualize the solution. If an object needs to be both flexible and stiff, the Table of Contradictions brings us directly to a visual possibilities for solutions. For instance, if an object must be both sharp and blunt, visual images can be created directly from the application of suggestions from the table.
The Table of Contradictions is so rich with possibilities for solutions that the Contradiction Matrix is not required. This can be illustrated by the well known example of driving piles to support piers. In order to drive the pier into the seabed, a pile driver is used. Driving the pile can be difficult when the seabed is dense or rocky. Following is the function which describes the problem. The improvement is ease of penetration.
Note that one way to drive the function to the extreme is to make the pile sharp. Unfortunately, a sharp pile continues to sink once a heavy structure is placed on top. The Physical Contradiction is that the pile must be both sharp and blunt. Please scan the Table of Contradictions in Breakthrough Thinking and note how many solutions to this problem are used as examples.
The first step to resolving the physical contradiction is to consider the various tools at a higher level. Four considerations are given when something else gets worse.

Reduce the Cost Penalty Often the contradiction arises that an improvement must be made, but the cost appears to get much worse. In this case, any new costly objects must take on additional functions. A good example of this is the recent introduction of solar panels that double as roof tiles. If a new house is being built, or an existing house requires re-tiling, the cost penalty of the separate systems are shared, thus dramatically reducing the combined cost.
Fix the New Offending Function If no solution can be found in the Table of Contradictions, identify the function that gets worse and begin the solution process again at Eliminating and Replacing Function Parts.
Introduction of New FunctionsSometimes, the only thing that gets worse is that while we know that a controlling variable must be driven to the extreme, we do not have a way in mind to make this happen. This requires the introduction of new functions. Since the function is known, we move back to the step of Eliminating and Replacing Function Parts for Useful Functions. Here we consider the ideal modification and the ideal tool to deliver this new modification.
Table of ContradictionsThe Table of Contradictions (Physcical) is the most common method for resolving contradictions. The contradiction is that a controlling variable must have a value (+) and an anti-value (-). In addition to solution steps, each of the methods in the Table of Contradictions gives an example and a diagram with contains pluses and minuses. For example:

The diagram is used by inserting the value and anti-value into the diagram in place of the pluses and minuses. For example, if the controlling value needed to be flexible and stiff, the diagram would be modified in this fashion:
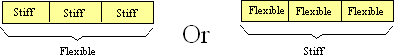
The number of methods for resolving contradictions is large. Usually, several of the solution methods will work. Take your time with each solution method. Work through the steps and consider ways to make it work. It is rare that a contradiction cannot be resolved by at least one solution method from the table. As mentioned, the pier driving solution is used in many places to illustrate various solution methods.
In classical TRIZ, the principal tools for resolving Physical Contradictions are separation in Space, Time, and Scale (Parts have anti-property of the Whole). The Table of Contradictions was mostly derived from these three separation principles and the 40 principles used to solve technical contradictions. A few tools were broken out of the Standard Solutions.
Four categories have been added.
Separation by Scale in Time: Allows for the contradiction to be resolved gradually as elements are added over time. A minimum of two elements are required
Separate in Direction Path or Plane: Here it is recognized that an object can occupy the same space and time and exhibit both contradictory properties. For instance, a flat pane can be stiff when the force is applied in one direction and very flexible when applied in another direction. The whole pane is both flexible and stiff in the same time and space.
Relative Measurement Standard: This method of solution also allows for an object to carry both conflicting properties at the same time and in the same space. An object floating in a liquid can be either heavy or light when compared to the density of the liquid it is floating in.
Field Susceptibility: Allows for an object to carry both contradictory properties at the same time, depending upon the field applied.
Expand and Consolidate To this point in the solution-process, the focus has been on one function at a time. Next, we consider expanding the solution to include more functionality or to consolidate elements. Tools were drawn from S-Field analysis, earlier version of ARIZ, and System Evolution Theory.
Expand the SolutionAt this point, the main disadvantage has been overcome. Now we look for ways that we can expand the solution out to other products or merge tools which perform different functions on the same product. The most important tools will be detailed here.
Combine Solutions One of the most important considerations is to combine some of the best solutions to this point. Often, the relative strength of each solution is not known. Rather than testing the solutions one-at-a-time, the solutions can be combined DOE style. As with any experiment, we stand to learn something of the physics in this step.

Merge Different Tools or Make Different Tools Interact Look at the several tools that operate on the same product. Can they be merged or made to Interact with each other. The example is given of wall anchors and drills. When a picture is hung, a hole is drilled in the wall and then an anchor screw is inserted. Both the drill and the screw operate on the wall. The wall, likewise, operates on the screw. Merging the drill and the screw makes the operation much faster and less complex to perform.
![]()
Merge the Anti-Tool Identify the opposite or anti-modification. Does a tool exist to perform the anti-modification? Combine the Tool and the Anti-Tool. If one does not exist, consider the possibility of creating one and then combining it with the Tool that performs the modification. The example is given of a common hammer which performs both drives nails and removes nails.

Consolidate the SolutionMost solutions are not as compact and efficient as they could be by this point. Consolidating elements that perform like functions reduces the number of elements.
Consolidate Multiple Product and Tool Elements If part of the tool or product can be shared between multiple elements, then consolidate the element. This is especially true when the solution involves multiplying Tool or Product elements. The example is given of a hammer and a pick which are combined. Two handles would be unwieldy and inefficient. The handles are merged.

Folding Elements Folding elements into each other makes the system more compact. Sometimes, unexpected useful benefits will emerge
Merge with Super-System and Consolidate One of the most important considerations is to merge with the super-system. Often, the super-system will have elements which perform duplicate functions. A house is part of the super-system for most refrigerators. What elements in the house are duplicated in the refrigerator? The structure and insulation are duplicated. If the refrigerator merges with the house, it can make use of the house structure and insulation.
Solution Branches Each decision point in this linear sequence of tools falls in a logical and natural order. The output of each step is required in order to move to the next. That output may be assumed or actively created. For example, consider a problem solver who wants to start solving an apparent contradiction with no other consideration. He or she has already assumed a critical function along with its attending parts (product, modification, effect and tool) as well as a controlling variable which has an extreme property.
The following figure shows the branching of the solution paths at each decision point for a Useful Function. (Branching is slightly different for Harmful and Informing functions). Each step allows for multiple decisions, with each decision forming a new solution path.

Solution Branches
As shown by the above diagram, dozens of practical solutions are possible and, in fact, occur commonly in practice. These solutions should be evaluated on the basis of ideality and business and customer needs.
Conclusion It is hoped that the reader will recognize that a separation and regrouping of tools is necessary to allow for the further advancement and growth of TRIZ. A linear sequencing of TRIZ tools has been presented that results in a branching solution path. The output of each step must be actively sought or presumed in order to proceed with the next step.
References
The Innovation Algorithm, Genrich Altshuller, Technical Innovation Center, Inc. 1999
Creativity as an Exact Science, G. Altshuller, Gordon and Breach, 1985.
40 Principles: TRIZ Keys to Technical Innovation, G. S. Altshuller, Translated by Lev Shulyak, Technical Innovation Center, 1997
Systematic Innovation, John Terninko, Alla Zusman, Boris Zlotin, St. Lucie Press, 1998
And Suddently the Inventor Appeared, G. Altshuller, Technical Innovation Center, 1994
TRIZ: The Right Solution at the Right Time, Yuri Salamatov, 1999
The Science of Innovation, Victor R. Fey, Eugene I. Rivin, TRIZ Group, 1997
Invention Machine Software (DOS Version to 1998 Tech OptimizerÓ)
Ó 2002, Larry K. Ball, all rights reserved.

