Editor | On 13, Jan 2006

By: Antonio Cesar Lettieri, Takashi Yoneyama

Su-Field: An Educational Example of
Inventive Problem Solving in Electrical Engineering
Antonio Cesar Lettieri
lettieri@directnet.com.br
Empresa Brasileira de Aeronáutica – EMBRAER S. A. – Brazil.
Takashi Yoneyama
takashi@ele.ita.br
Instituto Tecnológico de Aeronáutica – ITA – Brazil

ABSTRACT
The objective of this article is to present an educational example of a inventive problem involving only elementary circuit theory, easily solved by applying Su-Field analysis, but that constitute quite a formidable challenge to those who attempt to find the solution by looking only to the schematics.

1. Introduction
The way people normally react to inventive problems is described by:

• If an inventive problem is presented together with one solution, most people just do not believe that the problem is difficult. People usually tend to focus on the simplicity of one clever solution and are led to believe that finding it is simple. Moreover, they usually see the problem as so simple and obvious and do not see alternative solutions (ever when the alternative solutions exist)

• If an inventive problem is presented without any solution and sufficient time is allowed to look for a solution, people will eventually notice that it is not so easy to attain success by trial and error method. Then, when a clever solution is provided after some hard work, many will value the given solution. However, even in this case they may have doubts weather they would not find the clever solution by themselves and once more they will most likely not see alternative solutions.
In a recent issue of IEEE Potentials Magazine [1] , a very interesting conceptual problem in electrical engineering and involving only elementary circuit theory was proposed. With the aid of TRIZ, solutions were found for this problem quite rapidly, whereas some well-qualified electrical engineers could not succeed in a reasonable time. Because of the simplicity of the problem and the elegant way the Su-Field analysis can be applied, it was found that it could be of value for educational purposes in TRIZ courses.

2. The problem
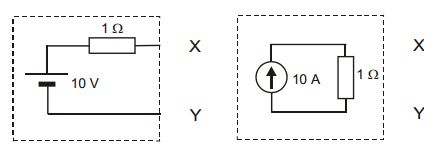
The problem consists in devising a test that allows distinguishing two identical black boxes, each containing one of the two following electrical circuits (Figure 1):

The circuit in the right is the Norton equivalent of the circuit in the left of Figure 1 (Thevenin circuit). The first circuit has an ideal voltage source in series with a resistor. The second circuit has an ideal current source in parallel with a resistor.
The test to distinguish one circuit from the other can use anything external to the box, such as resistors, capacitors, diodes, threads, wires, corrosive substances, etc… The only restriction is that the box cannot be opened.
When faced with this problem, most electrical engineers wonder if a solution could exist, since they have seen a mathematical proof that these circuits indeed are equivalent. This leads to a strong psychological inertia that precludes solving the problem. They usually try to put all sorts of linear and non-linear elements between X and Y in the hope of finding a solution.

Some engineers may even solve the problem, but usually remains locked in a single solution.

Using TRIZ, two conceptually different solutions could be found in a quick way and suggested the high educational power of this problem. One does not need to receive the solution ready. Instead he has only to learn a few basic elements of TRIZ to solve the problem alone.

3. The Inventive Solution
First one can intensify the restriction of the problem. Since it is allowed to add anything between X and Y terminals, one is allowed to use nothing.
In order to intensify the restriction, the circuits are redrawn as if the terminals X and Y were not accessible, so that one gets:

As the second step, one can state the problem by using simple words instead of technical jargon. In the present case:
“ We want to distinguish the Thevenin Circuit from the Norton Circuit”… is replaced by,
“ We want to distinguish two different electrical circuits”…which is replaced by,
“ We want to distinguish two different material objects”
Now one can use Su-Field Analysis. In this problem one has two substances:
1. S1 = Thevenin Circuit
2. S2 = Norton Circuit
Now, a Field is needed to complete the Su-Field (Inventive Standard 1-1-1). Fields that does not provide a direct solution to this problem, such as the gravitational field, acoustic field and radioactive fields can be discarded. What about the Thermal Field and the Magnetic Field?
By considering such fields one can quickly find two solutions: The first solution uses the thermal field. The two external points X and Y are open, so the Norton circuit will produce heat (Joule Effect) while the Thevenin circuit will not produce heat. One can just touch each box with a finger and determine which one produces heat.

The second solution uses the magnetic field. If the two external points X and Y open, the Norton circuit will produce a static magnetic field (the loop of current will produce magnetic field) while the Thevenin circuit will not produce magnetic field since the current flow is zero. The Norton circuit acts, therefore, as a magnet and one can distinguish the two circuits using a compass.
Both solutions are inventive solutions, which are conceptually different.

4. Conclusions
A simple educational TRIZ example for electrical engineers was presented. Students quickly found the two different conceptual solutions for a problem that they start believing as “impossible”. After obtaining the inventive solutions by themselves, the students reported that they could notice very clearly the concepts involved in TRIZ, and particularly in Su-Field Analysis, thus strengthening their confidence in the theory.

5. References
[1] Mack, D. R. (ed.) – Gamesman Problems: Problem 2. IEEE Potentials, fev/mar, v.23, n.1, pg. 47, 2004.
[2] Salamatov, Yuri (1999) TRIZ: The Right Solution at the Right Time Insytec B.V. – ISBN 90-804680-1-0

6. About Authors
Antonio Cesar Lettieri works as a Senior Product Development Engineer at EMBRAER S.A – Empresa Brasileira de Aeronáutica. He graduated in Electronics Engineering in 1981 (at ITA). He joined EMBRAER in 1984, after obtaining his Master Degree in Electronics Engineering (at ITA). International experience in technology transfer includes 4 years in Italy and 3 years in Israel. He started to be involved with TRIZ in 2004 and created a TRIZ introductory course at his company.
Takashi Yoneyama is a Professor at ITA – Instituto Tecnológico de Aeronáutica. He graduated in Electronics Engineering in 1975 and in Medicine in 1993. He obtained his PhD at Imperial College of Science, Technology and Medicine in 1983 (UK). He is interested in Technological Innovations and has co-authored a book on Digital Economy.