Editor | On 20, May 2003

By: Pentti Soderlin

pentti.soderlin@netlife.fi

One of the basic concepts in TRIZ is Ideality. It is closely related with the concept of Value of L.D. Miles in Value Analysis/Value Engineering. However some differences exist. Ideality has also been described in various sources in different ways, which do not always coincide or fulfil the need of basic arithmetic rules and are therefore less “scientific”. On Value in Value Analysis
The concept of Value in Value Analysis/ Value Engineering is to describe best value or “optimal” product. The Value[1] has been defined as

Value = Function/ Cost

Miles also has different kind of Values: that of Use, Esteem, Exchange and Cost. The latter is actually no Value, because the Function(?) is missing. The Figure 1 represents an imaginative or fictional situation where numerous product or service providers have the aim of fulfilling a need, functions. The optimal or best Value is the tangent (from the origo) of the Function – Cost curve.
If in the Figure 1 a number of manufacturers from A to E are trying to produce a product with equal functions, only one – E – is near the optimum and represents best value. The others have possibly made wrong choices – trade-offs – in their development work. Below the curve there are no feasible products. Anywhere else above the curve the products represent poorer value. It also shows that adding features or functions beyond the Optimal point leads as well to a poorer Value.

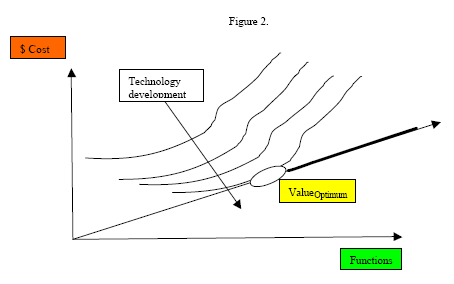
To image what happens within the time when technology and the ability of the manufacturers develop to produce the commodities better and cheaper and simultaneously the affluence of potential clients increases, see Figure 2.

The ValueOptimum changes its place downwards far right and to a better position. Again one or some companies are near the best Value. The Value ideality is only when the value curve is an asymptote (in bold) to the tangent. Cost may rise, but the value stays optimal.

On Ideality
The Ideality is often defined either as
1. Ideality = Sum(Useful Functions)/ Sum(Harmful Functions) [2]
or
2. Ideality = Sum (Benefits)/ (Sum(Expenses)+ Sum (Harms)) [2]
or
3. Ideality = (Perceived) Benefits/ (Cost+Harm) [3]
Although it is easy to understand that all of these formulas represent a symbolic equation as in Value
Analysis the Value concept
4. Value = Function(s)/ Cost
In the concept of Value all the functions are above the line. This gives to Value the dimension of
1/$
which means something although there is no whatsoever dimensions or scale of Function, but only the necessity of executing the required functions. Value Analysis is almost always cost driven. The very birth of the technique was to solve the problem of finding better and cheaper solutions for the intended functions. “Value for my money” as often heard from customers or “More for less” by Buckminster Fuller.
The equation 1. above gives the dimension of Ideality
1/1
which is acceptable. The Ideality is infinite when all the Harms disappear. The cost is excluded since we consider cost implicitly.

The situation is different with the equations 2. and 3. above. It gives to Ideality the dimension
1/(1+$)
which of course is a mongrel not to speak about the idea to include Time in the denominator [4] in Ideality definition.
The Harms or Harmful Functions are something like original sin. The technical system in itself carries always harms like the cost to manufacture or invest, the cost to operate and maintain, or simply things like pollution during the lifelong existence or the need of place and volume. If we can give a $ sign to each of these malfunctions, the equations 2. and 3. will work. However this is exceptional, since these things can be difficult to capitalize.
In [2] Savransky talks about Behaviors and Functions. The idea is that Useful Functions are positive and the Harmful Functions are negative in the “Arithmetic for Functions”. Harms will deduct the sum of Functions (horizontal arrow) and simultaneously add to Cost (verti cal arrow). Figure 3.

This pushes the Value curve upwards and deteriorates the Value. The “Real case” Value is lesser than the “Optimum Value” if all required positive Functions are to be fulfilled. Then the comparison of different competing products does not depend on the measuring of Functions. But the reality is not this simple: anybody who has tried to compare products or the tenders of different vendors in investment projects knows this. The re are always variations in the specifications whose cost are hard to estimate etc.

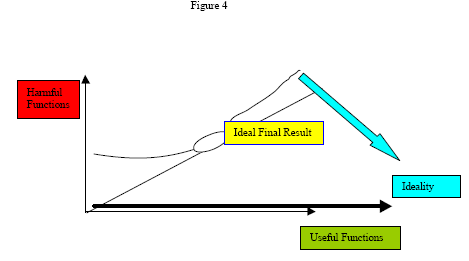
The ideal Value is a horizontal (bold) line with no cost but all Functions fulfilled and no Harmful Functions. At once we see that the Value is infinite, as it should be. The Best Value is aiming to the direction of ideality with less Harmful Functions. The required solutions are found “all by itself”, with no additional cost as it should.

The Ideality Curve
Somehow I would prefer the formula (1)
Ideality = Sum(Useful Functions)/ Sum(Harmful Functions)

presented by e.g. Savransky [2] . The quantity gets the dimension
1/1
which is more “scientific” than the rest. Savransky also gives a very good procedure from Ideality
towards Ideal Final Result, which is “the absolutely best solution of a problem for the given conditions”.
There are also in [3] figures to describe the Ideality as on S-curve. However, this type of S -curve is
usually presented to illustrate the product life cycle, product turnover and product profi tability. In the Ideality context it is not perhaps the most promising. The idea that in the Infancy/Growth period the Ideality would rise the most is somewhat dubious. Wouldn’t it better suit for the Maturity or even Retirement phase? If we think e.g. a car as an example, we can say that the car is in Mature phase and the cars certainly now much more nearer ideal ones compared with those in Infancy sometimes in the 1930’s or even earlier? But the definitions and again the shape of the curve are not exact ones and only illustrative?
If we emulate the Value curve from Figure 1., we get the Ideal Final Result and Ideality concept. The
trend goes along the blue arrow towards the Ideality.
Nothing “new” is introduced. The only change is that instead the Value definition we adopt the Ideality
for the same formula.

Discussion
1. Engineers are used to think everything in concrete terms. At worst they require calculus to matters or things which are nonmeasurable. So any symbolic equation requires additional explanations. It works if we do not try to calculate any values for them.
2. The Ideality is one of the key concepts in TRIZ. It very similar to the Value concept created by Altshuller’s contemporary Miles. But Miles didn’t think it to the extreme: Ideality. The analogy between these concepts is obvious.
3. In selling TRIZ services the cost consciousness could be significant selling point. Hence the Cost in equations (2) and (3) are understandable. There exists a Physical Contradiction: Cost should be present and Cost should not be present. Why not solve it the TRIZ way? Separate in Time, Space or Structure, or simply leave the Cost for Value discipline (Super System level)?
4. The Ideality definition (1) is more “scientific” and acceptable, but certainly not the definition (2), or (3) nor the one presented in [4] .
5. Next: Thoughts on Fields?

References:
[1] Lawrence D. Miles: Techniques of Value Analysis & Engineering. McGraw-Hill Book Co., London
[2] S. D. Savransky: Engineering of Creativity. Introduction to TRIZ Methodology of Inventive Problem Solving; CRC Press, Boca Raton, Florida, 2000
[3] Darrell Mann: Hands-On Systematic Innovation, Ieper Belgium, 2002.
[4] Anticipating Failures with Substance-Field Inversion by Thomas W. Ruhe, TRIZ-Journal, Feb. 2003.