Editor | On 16, Sep 2003

By: Darrell Mann
Director, CREAX nv, Ieper, Belgium
Phone/fax: +44 (1275) 342960
E-mail: darrell.mann@creax.com

Introduction
This article explores the nature and use of Asymmetry as a strategy for solving problems. It was inspired through a combination of interesting findings concerning asymmetry in nature during the ongoing TRIZ and biology research within CREAX. It also has its roots in thoughts about the presence of Asymmetry among the five contradiction elimination strategies in the TRIZ sub-set, SIT. This despite the fact that engineering experience would clearly indicate that asymmetry was nowhere near one of the top five strategies used in the successful elimination of conflicts and trade-offs.

The objective of the article is to describe an evolution trend towards increasing asymmetry in a wide variety of different types of systems. The article is divided into four main sections. In the first section, we introduce the increasing asymmetry trend through examples from natural, then technical and business systems. Secondly we introduce the concept of ‘matched asymmetry’. In this concept we suggest that the trend towards increasing asymmetry extends only to a point at which asymmetrical features of a system match the asymmetries that exist in the systems with which they are expected to interface. ‘Matched asymmetry’ should influence the manner in which we deploy both the increasing asymmetry trend of evolution and the Asymmetry Inventive Principle, and a third section of the article illustrates a simple case study in which we show strategies for identifying where external asymmetries exist or do not exist around a system. A final section draws together conclusions on the importance of Asymmetry in the overall problem solving context.

1) Asymmetry – Technology Evolution Trend
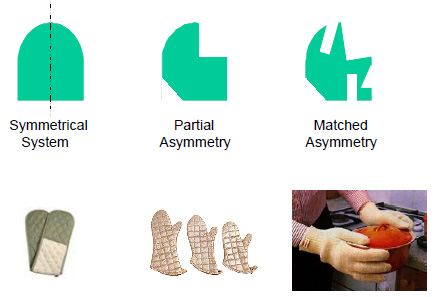
CREAX research studying the patent database has indicated the existence of a technology evolution trend related to the use of asymmetry (Reference 1). Specifically, the trend is one towards a matching of asymmetry to interface features between systems. The trend is particularly apparent in ergonomic design issues and the matching of constructed artifacts to match the natural asymmetries present in humans, but very definitely can also be observed in any other situation featuring external asymmetries. Figure 1 provides an illustration of the trend and an example of an ergonomics-driven asymmetry.

Basically the trend picture has been configured such that it operates in the same manner as the other TRIZ trends – i.e. benefits increasing from left to right across the trend, and thus, time also generally speaking travelling in a left to right direction.

Picking up on discussions elsewhere relating to the concept of evolutionary potential (Reference 2 for example), a system comprising symmetrical features – whether geometric, time-based or interface-based – when it’s surroundings feature asymmetries may be seen to possess unused evolutionary potential. This unused evolutionary potential may be used to create a better solution.

In the figure, the trend is drawn as a succession of three discrete stages. In the case of the oven glove example provided in the example we can see that these three stages have been followed in three different design generations. Evolution of asymmetry in relation to ergonomic issues can frequently be observed to pass through similar progressions. In many other instances – and particularly ones related to the evolution of natural systems – the evolution is more continuous in nature and it is far less clear that there are any discrete stages at all. As far as an Asymmetry Trend and this article is concerned, the main point is that systems can be observed to evolve towards some form of external asymmetry as either a continuous progression or in discrete stages. Ultimately in this case it seems that the distinction between discrete and continuous is not going to have a significant impact on the manner in which we use the trend.

Before exploring how in fact we will use the trend, this next section explores examples of where the trend can be observed and why it happens. We will start with examples from nature:

Asymmetry in Nature – From Molecular Through To Macro Level
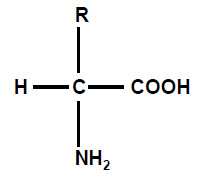
Natural systems are assembled from building blocks that are fundamentally asymmetrical in nature (Reference 4). Figure 1 illustrates the basic structure of the amino acids that make up the proteins that in turn form those building blocks. According to Reference 4 the fact that we see so many symmetrical things in nature given this asymmetrical start point is somewhat paradoxical. In many senses the ‘symmetry’ we observe is actually a mere approximation. All human faces, for example, are asymmetric to some degree. The fact that we generally find to find symmetrical features ‘attractive’ in others might begin to suggest how evolutionary pressures might cause symmetrical features to be favoured over asymmetrical ones.

At a higher level than amino acids, another major asymmetry in nature involve the fundamental differences between male and female. Reproduction – sexual reproduction at least – requires both sides of the asymmetry in order to happen. Although nature tends to favour approximations of symmetry constructed from these two fundamentally asymmetric foundations, there are many cases of natural systems evolving asymmetric forms. Reference 5 provides a broad-ranging spectrum of examples. In general we can observe nature evolving such solutions in order to solve contradictions. In the case of the fiddler crab – Figure 3 – we can see an extreme example of asymmetry in action.

The fiddler crab has evolved two massively different pincers as a way of allowing it to deliver the broadest possible range of functions – one large one for gripping and crushing; and a much smaller one for more delicate manipulations and access to small spaces.

Similarly, Tengmalm’s owl has solved a problem faced by several predators of the animal kingdom relating to how it locates its prey. Location is a particular problem in the initial stages before visual identification has occurred. Visual location in predators is generally limited to a relatively small field of view (in order that once visual location has happened, they can gather accurate depth of field and hence distance information using binocular vision). This limited field of view means that a large area can be tracked only by large head movements (which can make the predator easier to spot by the prey), or by using another non-visual means. In the case of owl’s the non-visual prey location means is auditory. The problem with listening for prey is that it is often very difficult to identify where in three dimensional space a sound is coming from. Humans are particularly bad, for example, at working out where sounds orignate – and the designers of emergency service vehicles everywhere have had to use many tricks to help the public identify which directions a vehicle is coming from.

Tengmalm’s owl on the other hand has evolved a rather simple solution to the acoustic location problem. By employing asymmetries (Figure 4) in the shape and position of left and right ear openings, it is able to exaggerate differences in time, loudness and phase of sound waves reaching the two ears, and thus far better able to establish what (elevation) direction a particular sound is coming from.

Asymmetry may be seen in nature as a means of using available resources to greatest possible effective. The fiddler crab could, for example, have evolved a pair of large pincers and a pair of small manipulators, but this would have required additional resource and added what would have been unnecessary complexity to the system. In general, the emergence of asymmetries like those found in the fiddler crab, Tengmalm’s owl and a plethora of other instances offer an effective means to resolve contradictions.

Asymmetry in Technical Systems
Probably one of the most well known (or well used) examples of asymmetry in technical systems is the engine intake design of the Boeing 737 airliner shown in Figure 5. The 737 has acquired this intake shape in order to resolve a contradiction: When the airliner was first launched on to the market it featured an engine with a relatively small diameter, and so the wing and undercarriage design was fixed to allow adequate ground clearance for this particular engine. When the aircraft later came to be re-engined with higher efficiency, highbypass ratio engines, the diameter of the engine became greater. This meant that there was now insufficient ground-clearance. The problem could have been solved by re-designing the wing and undercarriage, but this would have meant very significant and expensive design changes. The incorporation of the asymmetric intake, on the other hand, offered a much cheaper solution. Asymmetry was used in this design to solve a conflict between area (of the intake of the engine) and length (the fixed height of the wing and undercarriage).

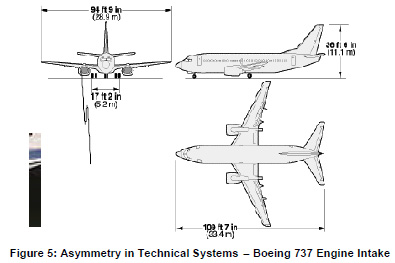
Another way of looking at this problem is that when the new large diameter engine was fitted, the ground beneath it became an ‘external asymmetry’ that necessitated the introduction of an asymmetry within the system.

To a large extent the asymmetry solution used on the 737 is not one that should really be classified as a very ‘strong’ one. The engine components rotating inside the engine fundamentally describe a circular path and so an asymmetric entry into that path by necessity creates turbulence effects that detract from the overall efficiency of the system. The use of asymmetry has generated a solution that, although it has increased the net ideality of the system slightly, has to a large extent also shifted the design trade-off from one position to another. That issue aside, the main point as far as this article is concerned is that the engine intake became asymmetric upon the introduction of an external asymmetry.

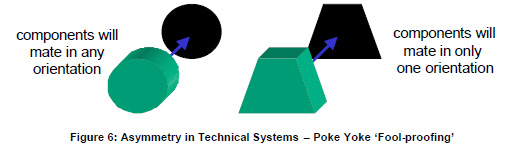
In other technical instances we can observe the introduction of asymmetries for reasons other than the presence of external asymmetry. In the case of poke yoke ‘fool-proofing’ designs for example – Figure 6 – asymmetries are introduced in order to ensure that a pair of components can only be joined correctly. In this case, the asymmetry has been deliberately introduced to generate a useful result and create an external asymmetry for the other mating component.

Whereas the introduction of this kind of poke yoke asymmetry was done at the expense of increased manufacture cost (traditionally because it has been cheaper to make a circular hole than a non-circular one), we increasingly see the cost-increase contradiction being resolved by novel manufacture methods. This is again a typical evolution path – introduce something that conveys a functional advantage, but at the expense of increased cost; and then resolve the resulting benefit versus cost conflict.

In addition to asymmetry being introduced to technical systems in order to match external asymmetries or create external asymmetries, recent research (Reference 6) has highlighted the widespread use of asymmetry to achieve aesthetic benefits. Here another form of ‘external asymmetry’ is at play. Take the two pictures, A and B in Figure 7 below and decide which one of them you prefer.

Chances are that you have picked picture B. Somehow it seems to convey a sense of movement and stronger sense of compositional balance. The difference between the two otherwise identical photos is that B takes account of the ‘rule of thirds’ and A does not. The external asymmetry here is that the human eye aesthetically prefers images in which the subject falls on or close to lines that divide the frame into even thirds. We can see that B does this in both horizontal and vertical directions, while A manages it in only the vertical direction.

This ‘rule of thirds’ concept is the external asymmetry in this case. In some senses the concept is in conflict with our preference for symmetrical faces, or in this case objects – if the ball of dung under the beetle was not round for example, or the beetle appeared asymmetrical in some way. One way of looking at this apparent conflict is to recognize the different perspectives present when we look at a system and a photograph composed with that system in it (i.e. when we take a super-system perspective). In any event, the point as far as the article is concerned is that there is an aesthetic preference for images which are asymmetrical. This preference is the ‘external asymmetry’ that images evolve to match in situations where aesthetics is important.

Asymmetry in Business Systems
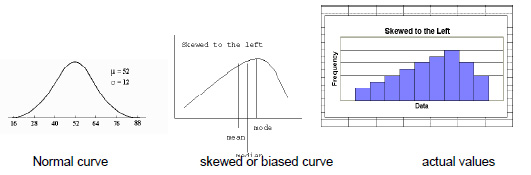
Asymmetry in business systems, although not as commonly applied as a conflict resolution strategy as it is for technical systems, can nevertheless be observed relatively frequently. Reference 3 contains a number of such examples. A very simple case from this source is the use of skewed distributions when trying to model situations. The ‘increasing asymmetry’ here involves, for example, recognizing that the seemingly ubiquitous normal curve very often fails to adequately model what the data in a system actually represents. As shown in Figure 8, the increasing asymmetry trend shifts from the symmetrical ‘normal’ curve to a mathematically skewed ‘partially asymmetric’ approximation to a point-to-point, fully modeled version of the actual data.

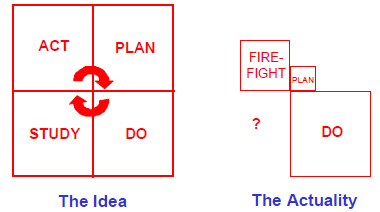
In a related vein, we may also see the Deming ‘plan-Do-Study-Act’ as a symmetrical system in which the area denoting each of the four different activities was intended to indicate the relative importance and amount of effort required at each stage. As illustrated in Figure 9, the actual time and effort split between the four activities in most organizations is somewhat different from Deming’s intention. We might think of this actuality as a non desirable asymmetry. Alternatively, we could use asymmetry in a more pro-active sense by recognizing that it is possible to bias any four of the PDSA activities to suit the need at any given time.

No doubt we could devote more time and space to describing more examples of asymmetry in business, technical or natural systems. Hopefully the desired point about the use of asymmetry and the existence of the trend has been made, and so we can move on to examine the idea of ‘matching’ the degree of asymmetry exhibited by a system to some
outside requirement.

2) Matched Asymmetry
Inventive Principle 4a, Asymmetry, describes the introduction of asymmetries and Principle 4c extends this to say ‘if the object or system is already asymmetrical, increase the degree of asymmetry’. The Inventive Principle, in other words, implies that the increase in asymmetry is a progression that doesn’t necessarily know any end. If we consider further increases in the asymmetry of the finger and thumb shape of a glove beyond where they are today, we can be reasonably certain that such a design will not offer wearers any additional benefit over the current hand-matched design. Hopefully this simple example serves to suggest that there are limits to how far the ‘increasing asymmetry’ direction is likely to travel before the advantages begin to turn into disadvantages.

Again in the case of the oven glove example in Figure 1, the evolution stages from left to right have increasingly matching the system to the asymmetries of the hand offers the user increasing dexterity when it comes to manipulating hot items. As soon as they reached the same level of asymmetry as the hand (‘the external feature’), there was no longer any point in becoming even more asymmetrical. The important issue, then, in determining how far a direction towards increased asymmetry will travel, is what external asymmetries exist. The key question when trying to locate external asymmetries is ‘is there a genuine and repeatable asymmetry for my system to match to?’ In the case of the oven glove, the repeatable asymmetry is that every human hand (disabilities excepted) has certain common features – four fingers, an opposing thumb, a left and a right – that a designer can safely try and match the system to.

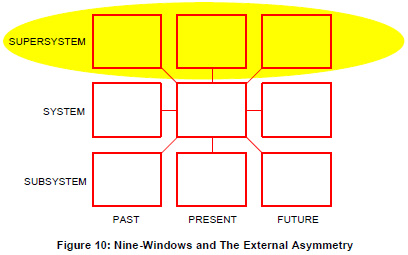
In the case of pens and other writing implements, the ‘genuine and repeatable asymmetry’ is not present since different people hold the pen in different ways and, more specifically, a proportion of the population is left-handed. Left-handed people would find it difficult to use implements designed solely for right-handed use. This is why most writing implements are largely symmetrical (or rather why there are a number of specialist ‘left-handed’ pens on the market to compensate for the fact that several sectors of the pen industry have assumed that they should bias their designs to suit the 90+% of the population that are unfortunately righthanded). Once we define our system as ‘pen’ or ‘oven glove’, or whatever, the key to locating the external asymmetries involves thinking about the things around that system. In this regard, the 9-Windows/’system operator’ concept illustrated in Figure 10 can be helpful. Identifying the external asymmetries demands an exhaustive study of all of the things that exist in the super-system around the system we have defined. As suggested by the figure, it is not simply a case of looking to the super-system in the present in order to identify those external asymmetries; rather we ought also to examine whether those asymmetries that might exist in the present will continue to exist throughout the defined span of the past-present-future time axis.

Thus, to take a simple example, we might think about the benefits that might accrue from incorporating asymmetry into the humble golf-tee. Certainly, if we construct an evolutionary potential plot of the current design of tee, we will identify asymmetry as an untapped resource (Reference 7). Somewhere, asymmetry ought to allow us to improve the ideality of the tee. If there is an external asymmetry we can find. In the next section we will explore how and where we might do this.

3) Matched Asymmetry Example – Finding the External Asymmetry
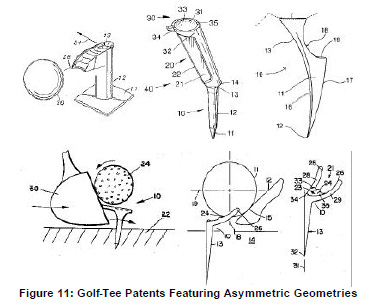
Finding genuine asymmetries is often not as easy as it first appears. Figure 11 below, for example, illustrates a number of granted golf-tee patents where the inventor has assumed that there is a benefit to be obtained by introducing asymmetries into the tee design:-

What is the problem with all of these designs? Answer; they have assumed that an external asymmetry exists, when in actual fact it doesn’t. At least not at all of the time-frames suggested by the 9-Windows. Certainly, during the time when the ball has been teed and is awaiting a strike from the golf-club, there is an asymmetry since we know that the club is always going to arrive from a certain direction. But this is not the whole story. During normal use of a golf-tee, the golfer picks up the tee between two fingers, picks up the ball with all of the fingers, manipulates the ball so that it sits in the cup of the tee, then uses the palm of the hand to press on the ball so that it in turn drives the tee into the ground. At no stage during this operation does the golfer have to worry about the orientation of the tee. All of the above designs, on the other hand, require a conscious effort by the golfer to align the tee to achieve the required position. The reason none of these tees has succeeded on the market is because they have all served to make life more complicated for the golfer – the process of spiking a tee into the ground being transformed from an operation that requires no conscious effort to one that, in the case of several of the designs, requires significant effort. If we determine that one of the constraints on the design is that it should not be any more complicated for the golfer to use (i.e. the ‘super-system, past’ box) then when we then examine the Asymmetry trend we need to take due account of this fact. None of the above designs in fact meet this constraint. This is because in each case, the golfer has to incorporate the additional function ‘align the tee correctly’ when inserting it into the ground. The fact that all of the Figure 11 designs have added this increased difficulty of use probably goes a long way to explaining their commercial failure.

The failure of Asymmetry in these examples, however, does not necessarily mean that Asymmetry cannot be used, merely that the above inventors have failed to use it effectively. All of them have identified and tried to exploit the presence of a certain amount of asymmetry – for example the golf-club will hit the ball from one direction and hence have tried to use the direction of the club to encourage the tee to stay in the ground after the shot has been completed (i.e. they have tried to eliminate the ‘lost tee’ problem).

They have found one external asymmetry (golf-club direction), but have failed to record the absence of another (the way the user holds and places the tee).

So, does this mean that there is no opportunity to exploit asymmetry in the design?
Answer; no. But it does mean that we need to adopt a different strategy if we are going to achieve a successful solution.

A smart design would recognize here that there is a need here for ‘asymmetry and noasymmetry’;
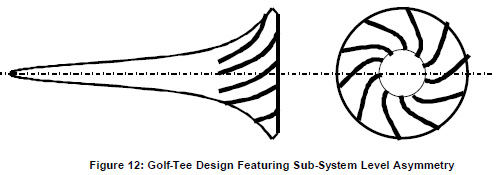
we want to take advantage of the golf club direction, but don’t want to complicate life for the golfer. It is a physical contradiction. A smart design would take advantage of the genuine asymmetry and have no adverse impact on the areas where there is no asymmetry. The physical contradiction resolution strategies can help us to resolve this problem. The design shown below, for example, has used the ‘transition to the sub-system’ strategy to produce a design which is symmetrical and asymmetrical – it is symmetrical as far as the golfer is concerned and is thus used in a manner identical to a ‘normal’ tee, but it is also asymmetrical as far as the club-head is concerned – the idea being that as the club head strikes the tee, it will deflect and rotate (if we’re smart, at the same time also driving the tee further into, rather than out of the ground – so we don’t lose it).

Thus we have found a means of employing asymmetry in a way that does deliver additional benefit to the user, without adding harm. If we are smart about the way we manufacture the Figure 12 design, we should also find there is a zero impact on cost.

Far more important the realization of a novel tee design is the identification of a strategy for incorporating asymmetry that can be deployed in other situations where an external asymmetry is not always present. Put simply, that strategy involves recognizing that a physical contradiction emerges (‘I want asymmetry and I can’t have asymmetry’), and that it is most effectively solved by transitioning to the sub-system. This turns out to be a strategy that so far, wherever we have applied it, it has given us an effective solution.

Conclusions and Final Thoughts
In summary, the Asymmetry solution triggers contained in TRIZ can help us to generate some very elegant solutions. The key to effective use is to identify the presence of external asymmetries. No external asymmetry means no benefit in introducing asymmetry within a system. External asymmetries often exist. The great asymmetry skills involve first finding them, and then identifying ways of isolating them from things that are not asymmetrical. When we can find external asymmetries that are sometimes there and sometimes not there, we can still make effective use of asymmetry by transitioning its use to the sub-system. One of the great things about using Asymmetry is that it often requires no additional use of resources. This may well be one of the reasons that nature makes such effective use of it. It might also explain why the trend of evolution towards increased use of asymmetry exists – benefits increase, while manufacture cost is effectively unchanged.

References
1) Mann, D.L., ‘Hands-On systematic Innovation’, CREAX Press, 2002.
2) Mann, D.L., Dewulf, S., ‘Evolutionary Potential In Technical and Business Systems’, TRIZCON 2002, St Louis, April 2002.
3) CREAX Innovation Suite 3.0, www.creax.com
4) Neville, A.C., ‘Animal Asymmetry’, The Institute of Biology’s Studies in Biology No. 67, Edward Arnold Publishers, London, 1976.
5) http://www.biology.ualberta.ca/palmer.hp/asym/asymmetry.htm#Small+Large
6) Mann, D.L., Dewulf, S., Zlotin, B., Zusman, A., ‘Matrix 2003: Updating the TRIZ Contradiction Matrix’, CREAX Press, June 2003.
7) ‘Constraint Management’, CREAX newsletter, www.creax.com, June 2003.